Particle dynamics in flows
Advection, aggregation and fragmentation dynamics of inertial particles in flows
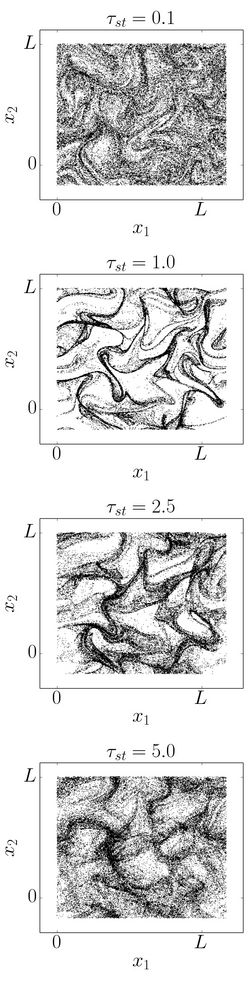
We analyze the motion and interaction dynamics of particles suspended in a fluid flow, where the flow can be air or watter. We are particularly interested in systems where these particles have properties, such as mass (density), which differ from that of the elements of the carrying fluid. In this case they are know as inertial particles, and their dynamics is of great interest for different research fields, from environmental to engineering sciences. Examples of such inertial particles can be rain droplets in clouds or sedimenting marine aggregates in the ocean. These particles do not follow exactly the flow field due to the action of several hydrodynamic forces. The action of these forces results in an inhomogeneous distribution of inertial particles, in other words they segregate into specific flow regions -- dynamically evolving attractors. The shape of these attractors will depend on the type of the flow field (convection flow Fig. 1 and turbulent flow Fig. 2), and on the properties of suspended particles (Fig.1 and Fig. 2). Additionally the presence of inerta makes particles near each other acquire significantly different velocities, which increases even more the number of collisions among them. We are interested in how these effects affect aggregation and fragmentation process in flows. To that extent we follow individually each particle to detect possible collisions, which result in aggregation events. On the other hand fragmentation can result from forces exerted by the flow on the aggregate (Shear force) or due to the aggregate reaching a limiting size. The dynamics evolves until aggregation and fragmentation balance each other, resulting in a characteristic steady state size distributions of aggregates. This size distribution changes with flow properties and fragmentation mechanism.

![[Translate to English:]](/f/5/_processed_/3/2/csm_ICBM-Logo-transparent-_91fe1c6774.png)